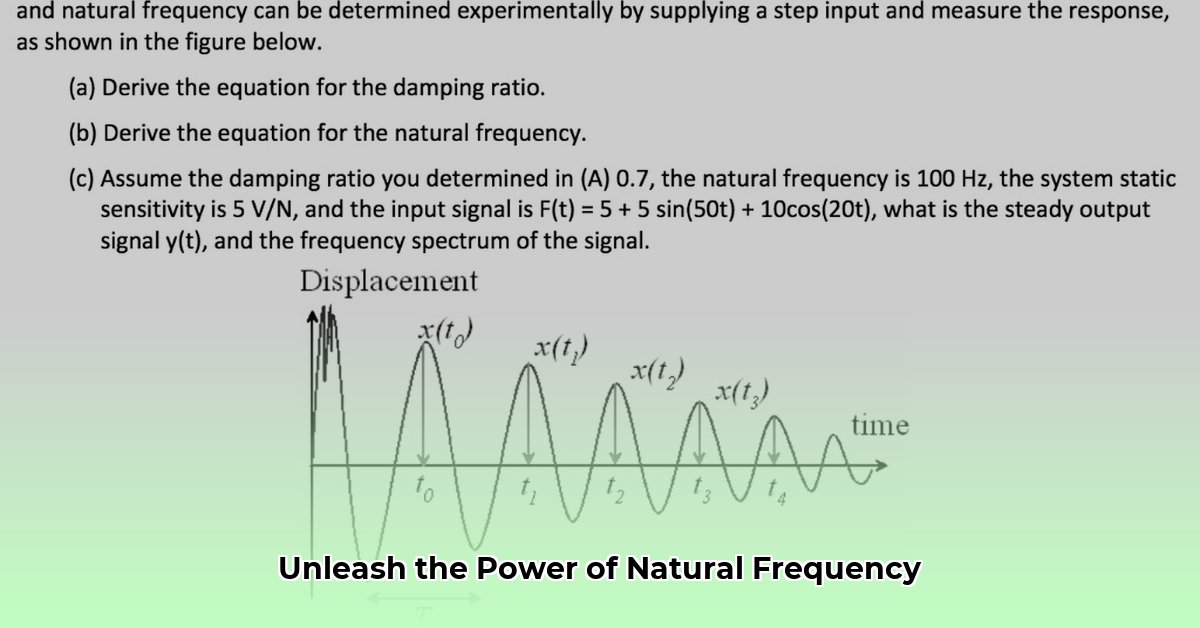
What is Natural Frequency?
Imagine pushing a child on a swing. You instinctively time your pushes to match the swing’s natural back-and-forth rhythm. That rhythm is its natural frequency – the rate at which an object vibrates when disturbed and then left alone. Everything has a natural frequency, from tiny atoms to massive bridges. This inherent property determines how objects respond to external forces and plays a crucial role in various fields, from music to engineering. This isn’t about how we make something vibrate, but how it wants to vibrate all by itself. It’s important to distinguish natural frequency (the object’s inherent vibration tendency) from forced frequency (the frequency of an external force) and resonant frequency (the frequency at which the object vibrates with maximum amplitude when driven by a force).
The Formula and Its Secrets
The natural frequency (f), measured in Hertz (Hz) or cycles per second, can often be calculated with a surprisingly simple formula:
f = (1 / 2π) * √( k / m )
Let’s decode the variables:
f(Natural Frequency): This tells us how many times the object vibrates back and forth in one second. A higherfmeans faster vibrations.k(Spring Constant): This measures stiffness. Think of a rubber band: a thick one (higher k) is harder to stretch than a thin one (lower k). It’s measured in Newtons per meter (N/m).m(Mass): This is simply how much “stuff” the object contains, measured in kilograms (kg). Heavier objects tend to vibrate slower, leading to a lower natural frequency.
Angular Frequency (ω)
Closely related to frequency is angular frequency (ω), measured in radians per second. Imagine the oscillating object moving in a circle. Angular frequency tells us how fast it rotates through that circle. The relationship: ω = 2πf or ω = √(k/ m).
Calculating Natural Frequency: A Step-by-Step Guide
Let’s illustrate with a simple example. We have a 0.5 kg weight attached to a spring with a spring constant of 100 N/m:
-
Identify the variables: k = 100 N/m and m = 0.5 kg.
-
Plug the values into the formula: f = (1 / 2π) * √(100 N/m / 0.5 kg)
-
Calculate: f ≈ 2.25 Hz. This means the weight will bounce up and down approximately 2.25 times every second.
Finding the Spring Constant (k)
Determining k often requires an experiment. Apply a known force (F) to the spring and measure the displacement (x). Hooke’s Law, F = -kx, helps calculate k = |F/x|. Plot force against displacement; the slope of the line gives you k.
Natural Frequency in the Real World
Natural frequency isn’t just theoretical; it influences countless real-world scenarios:
- Musical Instruments: A guitar string’s natural frequency determines the pitch of the note it produces. Tuning a guitar involves adjusting string tension to change their natural frequencies.
- Bridges: The Tacoma Narrows Bridge collapse tragically highlighted the destructive power of resonance. Modern bridges are designed to avoid natural frequencies that might align with wind or traffic vibrations.
- Earthquake Engineering: Buildings are designed to withstand earthquakes by ensuring their natural frequencies don’t match typical earthquake frequencies. This prevents resonance, which could lead to structural failure.
- MRI Machines: MRI (Magnetic Resonance Imaging) uses magnetic fields and radio waves tuned to the natural frequencies of atoms in our bodies to create detailed images of internal structures.
- Car Suspension: A car’s suspension system is designed with a specific natural frequency to absorb bumps and provide a smooth ride. Shock absorbers dampen oscillations, preventing excessive bouncing.
Beyond the Basics
Damping and Forced Oscillations
Real-world systems often experience damping– a resistance to oscillation that reduces vibration over time (like a swing gradually slowing down). Forced oscillations occur when an external force drives the system to vibrate at a specific frequency. While these factors add complexity, the natural frequency formula remains a fundamental building block for understanding more intricate scenarios.
Advanced Methods for Complex Systems
For complex structures like airplane wings or machine parts, more sophisticated methods are used:
- Modal Analysis: Identifies the individual natural frequencies and mode shapes (vibration patterns) of a structure.
- Finite Element Analysis (FEA): Breaks down complex objects into smaller elements to simulate their behavior under various conditions, including vibration.
Common Mistakes and Troubleshooting
- Unit Consistency: Ensure consistent units (kilograms for mass, Newtons per meter for spring constant).
- Measurement Accuracy: Precise measurements of an object’s properties are essential for accurate calculations.
Ongoing Research and Future Directions
Researchers are continually exploring natural frequency phenomena. Ongoing studies investigate new materials and designs to control and manipulate vibrations. This may lead to:
- More efficient energy harvesting: Imagine devices that capture vibrational energy from the environment.
- Adaptive structures: Buildings that can adjust their natural frequency to withstand changing conditions, such as earthquakes or strong winds.
Conclusion
Understanding natural frequency is crucial for comprehending the behavior of physical systems. This guide provides a solid foundation, empowering you to explore the world of vibrations and oscillations, from the music of a guitar string to the stability of a skyscraper. Remember that the study of natural frequency is an evolving field with ongoing research that continuously refines our understanding. While the concepts explored provide a strong base, there is always more to learn. While much is understood, the exploration of natural frequency and its real-world implications continues to be dynamic and exciting.
